The US Treasury yield curve is inverted by >100bps. It’s the steepest inversion since the early 80s. And in relative terms, it is the steepest inversion we have ever had.


The one (and only) inference we can make from this observation with certainty is that markets are anticipating falling yields. To lock in current yields for as long as possible, investors are willing to pay a premium for long duration even if that comes with higher volatility exposure. I have written about the mechanics in the article below:
Markets are usually very good at this. Historically, every inversion has signaled a local top in interest rates. And there are very good reasons why it will likely happen again.
As you know from previous articles, I expect that we will soon get QE again and that will likely come in conjunction with lower interest rates. The current level of debt in the system and the demographic set-up indicates that the sustainable interest rate is much lower than the level today. The short end currently trades at 5%. The long end trades at 4%. As I have outlined before, I think 1.5-2% (ST) and 2-2.5% (LT) are the levels markets will likely settle for in the long run with the help of the Printer.
So, if I believe that yields will fall, wouldn’t the most obvious thing for me to do then to buy bonds?
In this article, I explain why the answer is no. I will argue based on three aspects:
Reinvestment Risk
Expected return and volatility compared to stocks
Sentiment & Positioning
Before I dive in, I would like to emphasize that I am not provide any financial advice here, I am simply sharing my reasoning about what I am doing. Everyone’s investment objectives are different. There can be very good reasons to buy bonds at the moment, for example if the idea is to park capital that will be required in the next couple of years. My reasoning is primarily driven by my ambition to generate outperformance against a hypothetical world portfolio consisting of all assets I could potentially buy weighted at their market valuations. Even if I think that bonds will generate positive returns from here, I simply believe that they will underperform stocks. So this is a relative bear case, not an absolute bear case.
Twitter is currently full with charts like this:

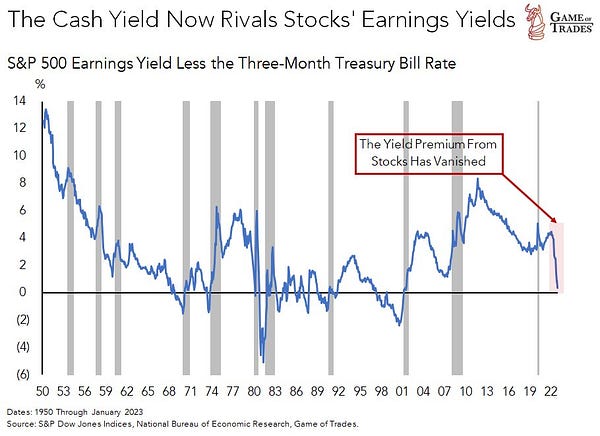

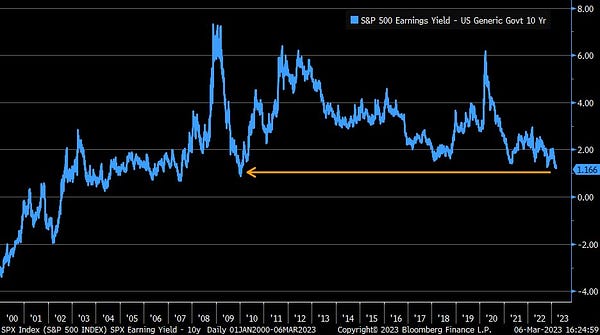
People compare the S&P earnings yield to treasury yields (typically anything between ultra-short term and 10Y). Then they observe that the spread is historically low. And then they conclude that stocks are too expensive when compared to bonds. This mental exercise is VERY flawed.
Reinvestment Risk
As I have indicated in the article below, it does not make sense to compare short/mid-term bond yields with earnings yields because of reinvestment risk.
This is particularly true during a severe inversion of the yield curve which signals falling yields down the road. The perpetual expected return of an investment into 6M, 2Y or even 10Y Treasury securities is significantly below where current yields are quoted.
In contrast to that, an equity investor gets the earnings yield of the S&P into perpetuity and it even offers a decent protection against future monetary debasement.

When the yield curve inverts and the equity risk premium tanks, this does not necessarily have anything to do with irrational exuberance. Markets are simply pricing in falling yields.
Locking in money today with the US Treasury for months or even years comes with an extremely high risk of having to reinvest that money at maturity either at much lower yields or having to invest it into equities at significantly higher valuations.
However, even if you agree with me on that, I can imagine you’d come up with a counter: Why not go into long duration then? Buying a 30Y for 4% should be quite a decent opportunity if yields will settle at <3% eventually?
Expected return and volatility compared to stocks
The problem with that is that reinvestment risk will be replaced by exposure to volatility that is in my opinion not warranted when compared to the expected return.
It depends a bit on the payout profile and the level of the coupon of each issue, but generally the effective duration of a standard 30Y semiannually paying coupon bond is 20. That means this 30Y coupon bond has an interest rate sensitivity that is comparable to a 20Y zero-coupon bond. There are some nuances, but generally duration means both weighted average timing of the cash flows and sensitivity to a 1% change in yields.
So, even if I intend to hold the bond until maturity, what does it help me to know I will likely get my principal back in decades if a simple 1% swing in the yield curve causes 20% or more volatility in my account? And even if I am willing to hold because I really don’t need the money until maturity, what about my vulnerability regarding monetary debasement? Just one repetition of what happened in 2020/21 and the purchasing power of my principal drops by 30-50%. I won’t recover from that with my 4% yield.
But isn’t the effective principal protection something to cherish at least? The US government is effectively default risk free. At least nominally I will get my money back with certainty. I don’t have that luxury with stocks, right?
Yes, theoretically you can lose money with the S&P over a 30y period. But since 1926 there has not been a single period like that. Not even close. The worst was from 1929 to 1958. And even then you would have returned >8% annually.
But at least the 30Y bond will provide more stable returns than the S&P, no?
Well, not really.
To illustrate that, I have built what I call a 30Y Treasury Total Return Index. I downloaded the daily yields on 30Y Treasuries here. Then I converted those into hypothetical bond prices assuming an effective duration of 20. And then I assumed that the investor will a) receive the daily valuation change and b) will on top of that compound daily at the quoted yields. The results look like this:


